HOME | DD
 FractalMonster — Not from the Mandelbrot Set4
by-nc-sa
FractalMonster — Not from the Mandelbrot Set4
by-nc-sa
#compass #exponent #formula #playing
Published: 2019-04-24 17:21:43 +0000 UTC; Views: 539; Favourites: 23; Downloads: 6
Redirect to original
Description
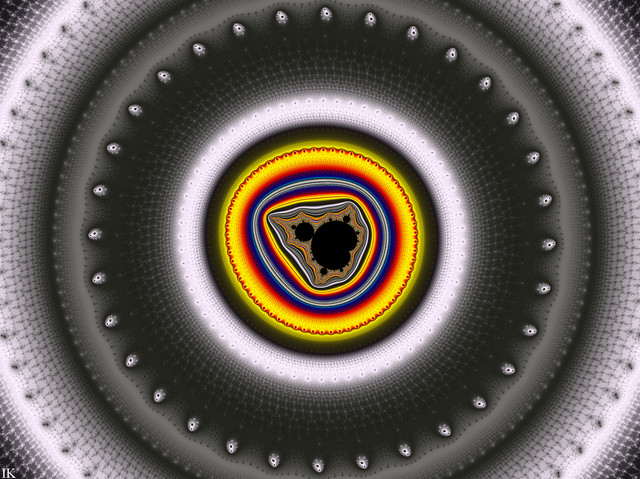
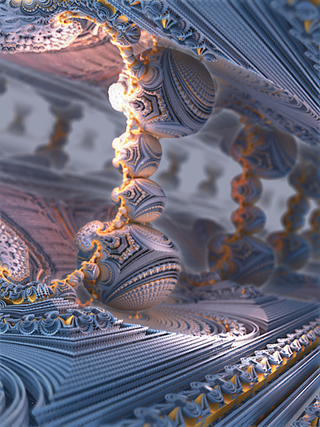
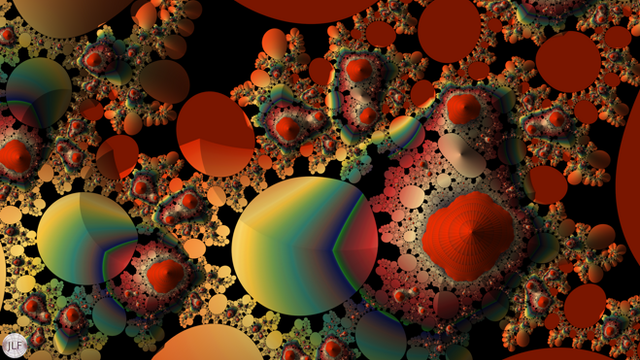
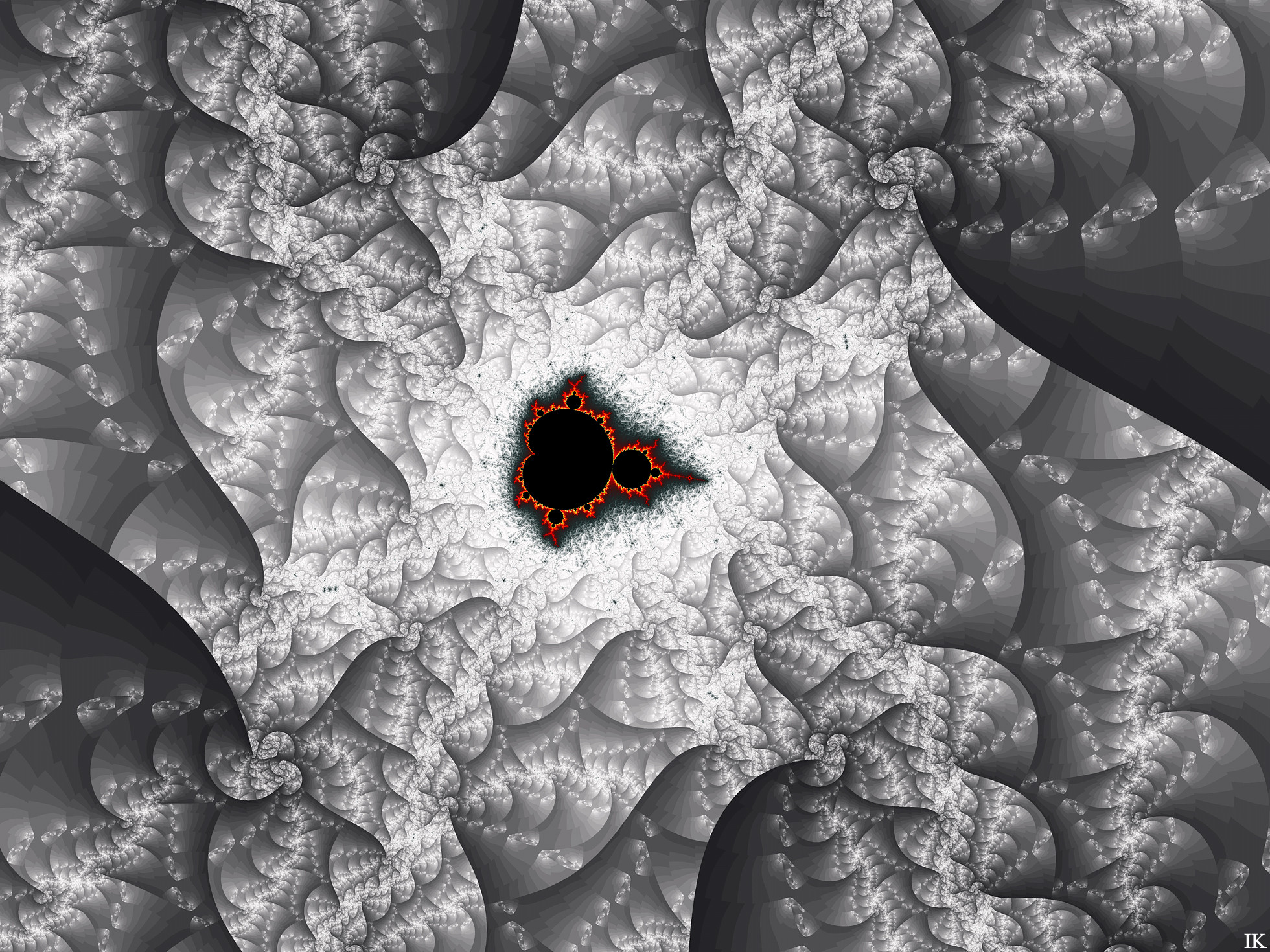
This one is a zoom-in into the tiny black dot visible in the upper left in the earlier deviationPlaying with the exponent in my compass formula, z -> z^d - da^(d-1) z. See my journal Fractal CompassesNow, my dear readers, I wanna promote the article,
27) Compasses
in my Chaotic series. Along with this journal there are four deviations uploaded,
Compass_d=2
Compass_d=3
Compass_d=4
Compass_d=5
The “d” is the exponent in the iterated polynom p(z) = z^d - da^(d-1) z, the a-plane plotted and “z” initialized to the critical point z = +a. Why this formula is called the “Compass formula”? Well, just look at the above deviations, especially for d = 3 and higher






 For d = 3 we actually have z^3 - 3a^2 z whic
For d = 3 we actually have z^3 - 3a^2 z whic 






The exponent "d" in this motive is set to 0+2i.
Software: Ultra Fractal.
Formula: Extended Compasses (adding a parameter "b". the full parameter space becoming a four dimensional hyper space).
Below the UF parametr file. Play and have fun







NotFromTheMandelbrotSet4 {
fractal:
title="Not from the Mandelbrot Set4" width=800 height=600 layers=1
credits="Ingvar Kullberg;4/13/2019"
layer:
caption="Background" opacity=100 method=multipass
mapping:
center=1.2617592572061/0.038907629385318 magn=4120.034
formula:
maxiter=10000 percheck=off filename="ik3.ufm"
entry="ExtendedCompasses" p_exponent=0/2
p_PlottedPlane="1.(a-real,a-imag)" p_hide=yes p_areal=0.0
p_aimag=0.0 p_breal=0.0 p_bimag=0.0 p_xrot=0.0 p_yrot=0.0
p_xrott=0.0 p_yrott=0.0 p_zrot=0.0 p_LocalRot=no p_diff=yes
p_bailout=10000000 p_dbailout=1E-10
inside:
transfer=none
outside:
density=0.1 transfer=linear
gradient:
smooth=yes rotation=-111 index=7 color=1709847 index=19
color=16579582 index=28 color=3026462 index=68 color=223 index=86
color=255 index=-235 color=57075 index=-24 color=16777212
opacity:
smooth=no index=0 opacity=255
}
Related content
Comments: 9

The web of gray around the red-outlined mandelbrot looks exceptional. Great job!
👍: 0 ⏩: 1


exponent not being a real number of 2 or greater ..
.. and thanks for the 
👍: 0 ⏩: 0

No problem
BTW, see you are a talent fractal artist 
👍: 0 ⏩: 1

A real beauty here too! Love all that silvery look!
👍: 0 ⏩: 1


👍: 0 ⏩: 0