HOME | DD
 FractalMonster — Hunting for something2
by-nc-sa
FractalMonster — Hunting for something2
by-nc-sa
#mandelbrot #set
Published: 2017-10-26 18:46:32 +0000 UTC; Views: 427; Favourites: 21; Downloads: 10
Redirect to original
Description
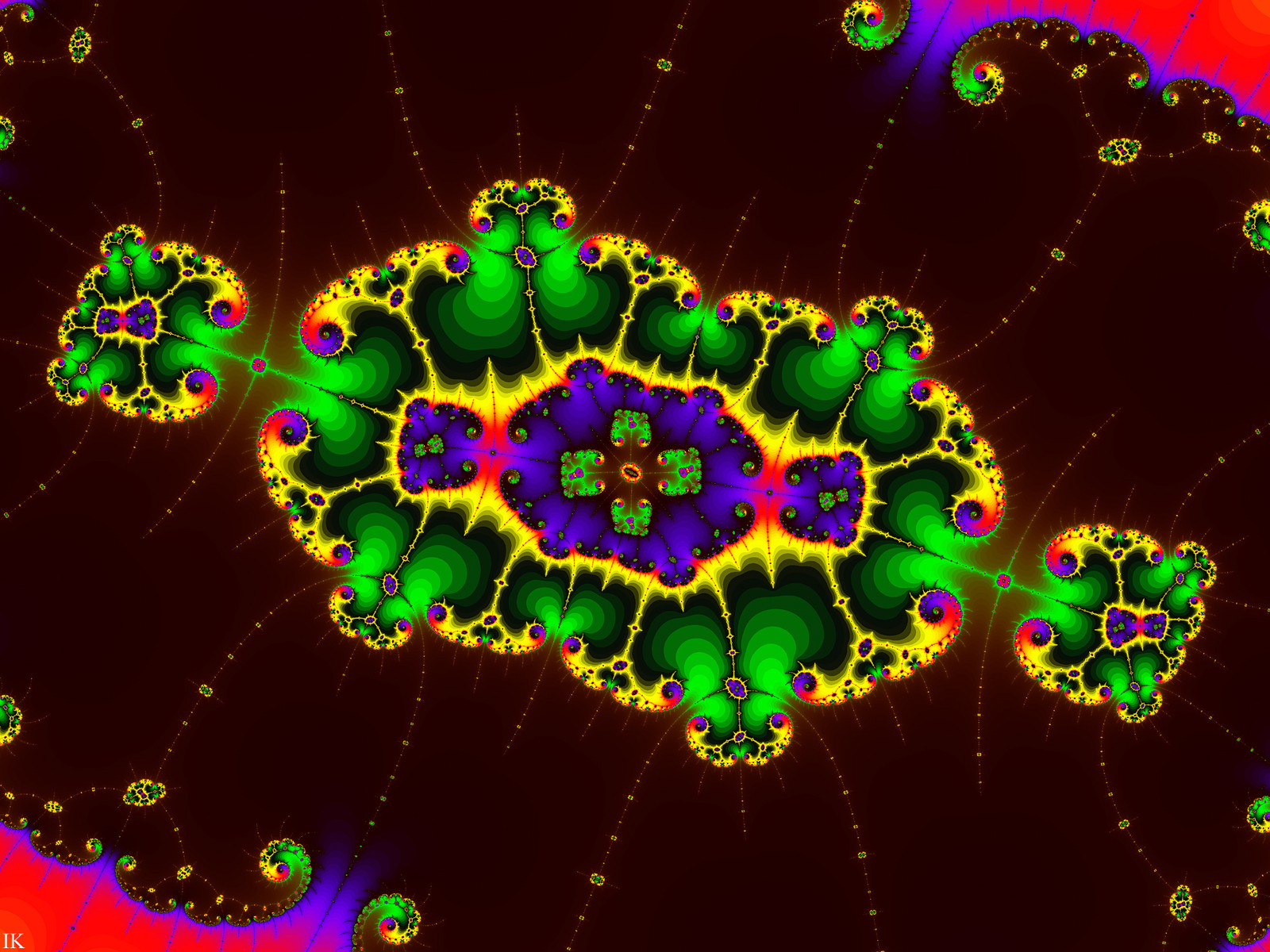
Formula: The standard Mandelbrot set.Software: Ultra Fractal.
For location and magnification, read the parameter file below (if you don't have UF)







Below the parameter file, play and have fun







HuntingForSomething2 {
fractal:
title="Hunting for something2" width=800 height=600 layers=1
credits="Ingvar Kullberg;10/22/2017"
layer:
caption="Layer 1" opacity=100 method=multipass
mapping:
center=-1.74894019183439270890095513429959349005/3.65012650954942090\
95636441852605993895e-6 magn=3.3917802E27
formula:
maxiter=10000 percheck=off filename="Standard.ufm"
entry="Mandelbrot" p_start=0/0 p_power=2/0 p_bailout=100
inside:
transfer=none
outside:
transfer=linear filename="sp.ucl" entry="Basic2" p_up=0.0 p_down=0.0
p_lp=2.0 p_lk=1.0 p_noblurr=no p_p=1.0 p_k=0.01 p_hightrap=0.0
p_lowtrap=0.0 p_mask=no p_solid=yes p_colindex=0.0
gradient:
smooth=yes rotation=-96 index=1 color=65530 index=57 color=329215
index=115 color=15335546 index=184 color=32 index=-94 color=17749
index=-54 color=62720 index=-22 color=461832
opacity:
smooth=no index=0 opacity=255
}
Related content
Comments: 10

This looks like the start of a cubic M-set filled with cubic M-sets.
👍: 0 ⏩: 1

That was what I actually was hunting for 


👍: 0 ⏩: 1

I was actually shocked to see that video as it's pretty much exactly what I did... in 2014.
Your idea may also turn out to be interesting. It's worth investigating.
👍: 0 ⏩: 1

You discovered the shape of the Cubic M set already 2014 
My idea? 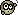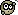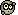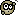
👍: 0 ⏩: 1

No, I mean I got the idea in January this year, then shortly after I discovered the video from 2014. I thought I had found something no one had done before and then I saw the thumbnail. I thought "WHAT!?".
I was not looking for this particular shape. I was looking for a way to show the evolution of only one type of shape at a time. In order to show the evolution of a shape as more morphings are applied, it needs to be copied (doubled) after each morphing. Doubling is in itself a morphing, which means you can alternate between 2 shapes, where morphing one automatically means doubling the other. The result is evolution of 2 shapes. The same can be done with 3 or more shapes, but how about just one? I wanted to find an elegant way to do it.
The first shape I tried it with was a tiling. My idea was "morph the tiling close to the center, then double it right at its boundary, following a row of tilings from the center" After a while the cubic M-set shape started to appear, albeit by far not as nicely. It was all distorted due to some mistakes and it wasn't even clear there were real cusps. I didn't think much of it but I liked what was going on, so I set another goal: finding a way to make the shape more symmetric, so I tried it the simplest way I could think of: building trees inside. It was only then that I noticed how close to the real cubic M-set shape it was getting and I was stunned. I couldn't believe it was something I found. I tried other shapes shortly after but they all ruined the symmetry.
👍: 0 ⏩: 1

OK, sorry I misunderstood you a bit 
You are probably the most systematic researcher of the hidden depth of the M set 
👍: 0 ⏩: 1

Nice to hear that UF will have perturbation, so I wonder of course how fast is it. I'm not expecting much. Ultra fractal was never extremely optimized.
And I think there is a misunderstanding again, lol. First I found the cubic M-set shape. Then I discovered the video. While the video is from 2014, I discovered it only shortly after the cubic M-set.
You should probably start using Mandel Machine. It has the speed required to zoom deep. Kalles Fraktaler is also very useful because of Newton-Raphson zoomen. Then you can design shapes. It's easier now than ever before.
👍: 0 ⏩: 1

Just installed UF6 and I don't manage it to work with old formulas written by Greenseng for higher paramaterspaces, so I have to have UF5 installed with UF6 side by side: Maybe I've done something wrong and there will be a solution. Edit: Fixed 
OK 


👍: 0 ⏩: 0